ISSN 0798 1015

Vol. 40 (Number 7) Year 2019. Page 19

Modeling of the cycle of reproduction process in the agrarian sector of economy (Ukraine)

Modelación del ciclo del proceso de reproducción en el sector agrario de la economía (Ukrania)

HUTOROV, Andrii O. 1; HUTOROVA, Olena O. 2; LUPENKO, Yurii O. 3; YERMOLENKO, Oleksii A. 4; VORONKO-NEVIDNYCHA, Tetiana V. 5

Received: 31/10/2018 • Approved: 12/02/2019 • Published 04/03/2019

Contents

1. Introduction

2. Methodology

3. Results

4. Discussion of results

5. Conclusions

Bibliographic references

1. Introduction

The economic life of society is organically connected with the processes of reproduction of all elements of the economic system: economic relations, their subjects, productive forces and economic mechanism. In its turn, economic development is characterized by a periodic change in the process phases, which is based on the cyclicity of the reproductive process.

Currently, the agrarian sector of the economy of Ukraine is a system-oriented segment of the national economy, where on average 13.4 % of gross value added was created in 2015-2017. The food security of the State, employment in the rural areas and in the processing industry, tax and other revenues to the budget depend heavily on the stability of its development. However, fluctuations in economic and market conditions, changes in weather and climatic conditions, the transformation of institutional support for entrepreneurship, globalization challenges and the dynamics of global trends in economic development lead to instability. Cyclicity of reproductive processes in the agrarian sector of the national economy leads to the emergence of a significant number of risks.

One way or another, the fulfillment of the tasks set by the Cabinet of Ministers of Ukraine related to the provision of the sustainable development of the agrarian sector and rural areas based on an innovative basis, to the guaranteeing of the country's food security, preventing over-production crises requires a well-considered countercyclical policy. In its turn, the effective anti-cyclical measures should be based upon the scientifically grounded economic and mathematical models. The allow to estimate the hidden periodicity of economic processes, to predict them in the short-and long-term perspective.

2.1. Framework

The theory of economic cycles, alongside with the theory of economic growth, belongs to the theory of economic dynamics, which explains the movement and the development of the national economy of a country. In economic theory, there are about two hundred concepts and theories of the causes of crises and their cyclicity. At the same time, up to this time, the nature of the cycle remains one of the most controversial and little-studied issue.

The industrial crises of the XIXth century, as well as the documented experience of previous agrarian crises, led to the organized research of these issues conducted by the classics of economic thought and, thus, to the formation of the theory of business cycles. Agrarian cycles were studied mainly from the point of view of fluctuations in prices for agricultural products, the dynamics of yields and the influence of solar activity on them. A detailed analysis of the genesis of cyclical economic development is given in the works (Aimar, Bismans & Diebolt, 2016; Pustovoit, 2016; Podlesnaya, 2017). One of the first computational schemes aimed to detect hidden periods in agroeconomic and agrobiological research is the method of linear selective transformations, developed by C. Buys-Ballot in 1847. According to him, if the trial period coincides with the actual period of the investigated process, then averaging on the segments of the initial time a series of length equal to the trial period does not change the value of the global period, while smoothing the components of other periodic perturbations (Buys-Ballot, 1847). On the other hand, the Buys-Ballot transformation is equivalent to the product of the output discrete process and the periodic sequence of impulses with the subsequent averaging of this value (Serebrennikov & Pervozvanskiy, 1965, p. 38).

It can be argued that the problem of detecting hidden periodicity in a discrete time- series belongs to the class of incorrectly set. In general, it reduces to the finding of such transformations of the source data so that it is possible to determine the parameters of the periodic components. In 1898, for the first time, A. Schuster suggested a comprehensive solution to this problem, when he substantiated the scheme of periodogram analysis (Schuster, 1898). In addition, the scientist introduced the notion of a phase diagram, which makes it possible to more accurately determine the frequencies that are approximated by the abscissa of extremums of amplitude diagrams. The use of phase diagrams in the periodogram analysis of economic processes simplifies the decision-making regarding the choice of this or that trial period as a global period of the seasonal component.

As a result of the research, O. Oliinyk also concluded that the most universal function for analyzing the cyclical vibrations of economic processes is the sinusoid, while accounting for the linear trend (Oliinyk, 2005, p. 67). Further studies of the cyclicity of the main reproductive processes in agriculture enabled O. Oliinyk to show that the linear trend can be replaced by any other trend equation, while the number of sinusoidal harmonics is advisable to limit to one.

According to literary sources, there are now more than 30 methods and approaches allowing to detect hidden periods (Serebrennikov & Pervozvanskiy, 1965; Kufenko, 2016). The main ones are linear transformations (Brooks’ transformation, Buys-Ballot scheme, ordinates averaging for the trial period), nonlinear selective transformations of poliharmonic processes (correlation methods, splines), periodogram analysis, Stokes’ integration method, singular spectral analysis, etc. In particular, we conducted a comparative analysis of the methods of periodogram analysis, Stokes’ integrals, autocorrelation functions, and Buys-Ballot analysis in terms of their relevance for detecting hidden periods in discrete time-series and assessing the cyclical nature of economic processes (Hutorov & Pogorelov, 2006; Hutorov, 2009). In addition, it was proved that in agroeconomic studies it is expedient to use several methods with further comparison and a critical analysis of the obtained results. This allows to define the durations of the global period and periods of higher order harmonics more accurately and with higher quality.

2.2. Economic aspect of simulation of cyclicity in the reproduction process in the agrarian sector of the economy

Some of the first characteristics of the reproduction cyclicity were provided in works of D. Ricardo. In particular, he noted that at different stages of society development, the capital accumulation has different speeds and depends on the productive forces of labor (Ricardo, 2001, p. 61). Not allowing the possibility of a crisis of overproduction, D. Ricardo did not fully consider the features of reproductive processes in the economy. At the same time, he generally justified the tendency of profit margins to decrease, thereby laying the foundations for K. Marx theory of industrial cycles.

Afterwards, in the early 1860's, C. Juglar showed the existence of medium-term economic cycles, lasting for 8-10 years. Their occurrence was determined by fluctuations in the volume of investment in the active part of fixed capital, the emergence of new types of technology (Juglar, 1862). At the same time, the increase in prices for capital goods leads to economic growth, and the decrease - to decline. In modern interpretation the very business cycle of Juglar has four phases (Podlesnaya, 2017, p. 41): recovery, expansion, recession and depression.

Thoroughly examining the capital movement, the mechanisms of its reproduction and the genesis of industrial crises in England, K. Marx concluded that the material basis of the cyclicality of the capitalist crises is the periodic renewal of fixed capital (Marx, 1969, p. 464-465). The duration of the business cycle suggested by K. Marx is 10-11 years, which is in general quite comparable with the results of C. Juglar.

In the context of studying the cyclicity of the dynamics of reproductive processes, the scientific contribution of M. Tugan-Baranovsky looks reasonable. Having analyzed different approaches to the essence of economic cycles, he concluded that the obstacle to sustainable economic and sectoral development is not so much exogenous factors, as the endogenous bifurcations of the economic system of different levels of the hierarchy, which also give rise to non-stationary processes. M. Tugan-Baranovsky, thus, proved the existence of cyclic laws in reproduction of the country's fixed capital (Tugan-Baranovsky, 1914, p. 285-305). In addition, the scientist has shown that the production cycle is explained not only by the periodic renewal of fixed capital, but also by the dynamics of market prices for the means of production and consumer goods. He explained the periodic capitalist crises by the inertia of investment activity, thereby outstripping J. Schumpeter (Schumpeter, 1939).

While considering various factors of reproduction, J. Kitchin in 1923 substantiated the micro-cycles of economic dynamics lasting for 40 months (Kitchin, 1923). They are related to the circulation of material goods, however, unlike the cycles of Juglar and Marx, they are based on the reproduction of current assets, the movement of inventories, the investment lag, caused by managerial decisions, information asymmetry, and so on. In addition, during the Kitchin cycle, there is no renewal of fixed capital.

In contrast to the cycles of reproduction of the active part of fixed capital and working capital, S. Kuznets modeled the periodicity of medium- and long-term economic dynamics, lasting for 15-20 years, through the reproduction of the passive part of capital goods (civil and industrial construction) (Kuznets, 1930). In his opinion, a function that describes the cyclic reproduction process should: have a certain boundary; reflect the decrease in the percentage rate of total output increase; to reflect the change in the absolute value of output due to influence of stimulating and de-stimulating factors; to show a decrease in the pace of growth while reaching the limit (Kuznets, 1930, p. 64). From the set of functions describing growth, S. Kuznets chose the Pearl-Reed (1) and Gompertz (2) curves:

2.3. Methods of estimating hidden periods in the dynamics of the reproduction process in the agrarian sector of economy

2.4. Research materials

Based on the above stated, we took the share of capital investment in gross value added in percentage terms as an indicator for studying the cyclicity of the reproduction process.

We fully share the opinion of M. Allais, the longer the study period (the “memory of the economic process”), the stronger and more stable the trend is, and the longer the global period is (the period of the main cycle) (Allais, 1998, p. 124). Our research covers actual data on Ukraine for 1956-2017, its source is the statistical yearbooks of the Central Statistical Office under the Council of Ministers of the Ukrainian SSR (1956-1986), the State Committee of the Ukrainian SSR on Statistics (1987-1990), the Ministry of Statistics of the Ukrainian SSR (1991), the Ministry of Statistics of Ukraine (1992-1996), the State Statistics Committee of Ukraine (1997-2009), the State Statistics Service of Ukraine (2010-2017). The data for 1918-1955 were not analyzed due to the peculiarities of the state creation of that time, the crises of the war and postwar years. This could’ve lead to a shift in the general trend and seasonal component. Information for 2014-2017 is given without taking into account the temporarily occupied territory and the anti-terrorist operation zone.

Gross value added for 1956-1990 was approximated by national income, taking into account the methodological comparability of these indicators. The data on the agrarian sector of the economy correspond to the “Agriculture branch” according to the All-Union Classifier of the branches of the national economy (1956-1991), the General Classifier “ndustries of the National Economy of Ukraine” (1992-1996), section “A” of the Classifier of Types of Economic Activities, 1996, and “Agricultural and Hunting, Forestry” (1997-2011), Section “A” of the Classification of Types of Economic Activities, 2010, "Agriculture, Forestry and Fisheries” (2012-2017). Since aggregations are generally the same, their analysis in dynamics is methodologically correct. No additional smoothing, filtering and interpolation of the time-series were conducted.

The fixed capital accumulation in Ukraine in 1956-2017 was uneven, especially during the reforms of the mid-1990's. In particular, in 1995-2004, even a simple reproduction in most sectors of the economy was not ensured. The stabilization of the national economy after market transformations intensified the reproductive processes, which affected the annual growth of net accumulation of fixed capital (especially industrial) in 2005-2009. However, the recession in 2012-2013 and the crisis began in 2014 led to a decline in the level of investment attractiveness, led to the outflow of capital from Ukraine and to a new stage of decapitalization and de-industrialization (Hutorov, 2017, p. 188).

The basis of fixed capital accumulation and its reproduction is capital investment. The priority of the choice of investment objects usually depends on the level of return on investment, which, in normal business conditions, corresponds to the dynamics of the norm of value added. That is, under the conditions of neoindustrial development, the objects of higher technological redistribution are more investment attractive, because they create more value. However, the export orientation of the agrarian sector on raw materials, its disintegration with the processing industry deformed the institute of investments, causing an excess of capital investment rather into the production of raw materials than into the food industry. At the same time, these capital investments are unproductive, their economic value does not correspond to the cost, as indicated by their volumes exceeding the gross accumulation of capital assets of the agrarian sector in 2013-2017. In other words, a significant part of capital investment does not lead to an increase in the capitalization of the industry, they are not mastered properly, the fundamental principles of reproductive processes are undermined. Given that GDP consists of household consumption expenditures, government consumption and investments, gross private investments and net exports, the dynamics of the share of capital investment in gross value added also seems to be critical.

Output data are discrete time-series, containing 62 members, the graph of which is shown in Fig. 1

Figure 1
Dynamics of the share of capital investments in gross value added in Ukraine in 1956-2020

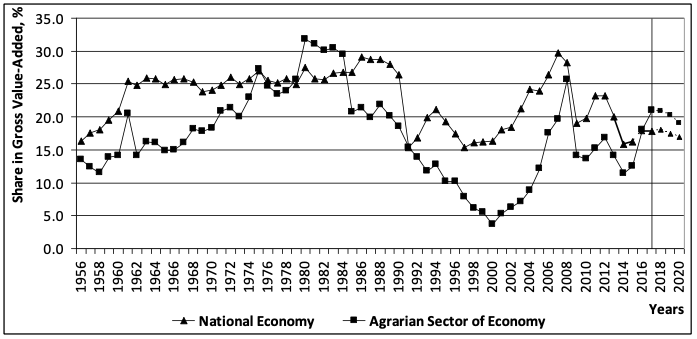
Notes. The data for the 1956-2017 are actual, for 2018-2020 – forecasted
(in the figure marked with a dotted line after the vertical one).
Source: author’ computations.

The discrete time-series of the share of capital investments in gross value added in Ukraine are characterized by a sufficiently large variation to put forward the hypothesis that they have periodic components. They are unstable relative to the average value
(Tab. 1).

Table 1
Descriptive statistics of the time-series of the share of capital
investments in gross value added in Ukraine in 1956-2017

Source: author’ computations

The absence of missed values allows the use general methods of statistical analysis without the need for additional interpolation. For data processing, we applied A. Hutorov's computer program “Expert system of “Periodograms”, which contains advanced algorithms for the search of hidden periodicities. During the calculations, the following basic parameters were taken into account: the maximum number of trial periods – 1000; the minimum value of the global period is 2; the maximum value of the global period is equal to the number of members in a row.

As a result of calculations, the hypothesis on the existence of periodic components of different durations in time-series was confirmed (Tab. 2).

Table 2
Characteristic of the periodic components in the time-series of the share
of capital investments in gross value added in Ukraine in 1956-2017

Notes. N. E. – national economy; A.S. –
is the agrarian sector of the economy
Source: authors’ computations.

Data of table 2 show that the reproduction process in the Ukraine has four distinct cycles: global (41 ± 1 year), medium term (20 ± 1 year), short-term (9 ± 1 year) and micro-cycle (3 ± 1 year). Mathematical models describing the periodic process are significant for Fisher's F-criterion at the confidence level of 0.99. Similar calculations for the dynamics of the share of capital investments in the gross value added of the agrarian sector of the economy show the existence of a global period lasting for 52 ± 5 years, a medium-term cycle of 26 ± 1 years, a short-term cycle of 13 ± 1 years and a cycle of 6 ± 1 years.

The decomposition of the time-series to the harmonics leads to a decrease in the statistical significance of the members of the higher orders harmonics as their number increases. In addition, this also causes the appearance of “parasitic” maxima on periodograms due to the displacement of the spectral density of power.

The period values got generally correspond to the normative terms of the use of fixed assets, while indicating the optimal time periods for reproduction cycles. In the agrarian sector of the economy, the increase in the average cycle duration is explained by the peculiarities of the reproduction of long-term biological assets, the influence of solar cycles on agricultural production, and so on.

To predict reproductive processes under the research, we used neural networks available in the StatSoft Statistica v. 10. The base neural network has been chosen a multilayered perceptron with architecture (6-2-1), with the Quasi-Newtonian algorithm for training of Broyden-Fletcher-Goldfarb-Shanno (BFGS), by the sinusoidal activation function of the neurons source and the identical activation function of the hidden neurons, as well as the projection window for 8 years. As a result of the training of 20 thousand neural networks during 20 thousand eras for each, two were selected with errors of 14.23 % and 15.89 % respectively. Besides, the control errors are 9.33 % and 9.87 %, which indicates a high level of reliability of 8-year forecasts. Forecasted in this way data for 2018-2020 shows that the short-term cycle with the ascending wave has been completed in 2017. Then, the period of investment and reproductive processes decline is expected both in the national economy, as a whole, and in the agrarian sector of the economy.

The resulting simulation cycles of the reproductive process in the Ukrainian economy and its agrarian sector generally correspond to the results of other scientists.

Thus, V. Podlesnaya gives data on the medium-term economic cycles lasting about 20 years (Podlesnaya, 2017, p. 324), as well as on long cycles of the reproductive process lasting 45-54 years (Podlesnaya, 2017, p. 323). The latter are caused by changes in technological patterns and the introduction of epoch-making innovations. The development of the national economy of Ukraine is characterized by the existence of micro-cycles, lasting 3-5 years (Podlesnaya, 2017, p. 419). According to O. Pustovoit, during 1997-2015 medium-term institutional cycle had place in Ukraine, it consists of two short-term cycles of 9 years (Pustovoit, 2016, p. 355).

The study of the crop yields dynamics in 1795-2007 and that of gross agricultural output in 1909-2006 allowed V. Rastyannikov and I. Deryugina to detect long 50-60 years cycles (Rastyannikov & Deryugina, 2009, p. 111).

According to I. Sokolov, I. Tarapatov and O. Borysenko, the cyclicality of grain production in Ukraine has the 14-years period (Sokolov, Tarapatov & Borysenko, 1996, p. 43). The results of the analysis of the dynamics of grain yields, sunflower seeds and sugar beets conducted by O. Oliinyk showed that they demonstrate short-term cycles of 15-18 years and micro-cycles of 2-6 years. At the same time, according to the scientist the cyclicity of solar activity is described by 10.5-11.0 years period (Oliinyk, 2005, p. 285).

According to I. Zagaytov, the duration of short-term reproduction cycles in the agrarian sector of the economy (Тс) is a function f(Ka) of the duration of the renewal of the active part of fixed capital (Ka):  where ΔD is the growth rate of real solvent demand in the inter-crisis period; ΔPr – increase in the productivity of the renewed capital (Zagaytov, 2011, p. 81-82). Thus, the scientist defined the average duration of short-term cycles at the level of 13 years.

The cyclicity of the reproduction process is an integral part of its dynamics, characterizing the evolutionary course of the development of productive forces, economic relations and economic mechanism within a country under the influence of global social transformations. These cyclical fluctuations are usually caused by natural-economic, socio-economic and institutional factors, and their material basis is the processes of social reproduction of capital in its broad sense. Therefore, all the “classic” economic cycles are organically linked with the investments and innovations, and a periodic innovation renewal is a necessary for the development of society and economy, in the long run it marks a change in technological modes. In our opinion, the micro-cycles of the reproduction process (as well as the Kitchin cycles) are due to the investment lag, while short-, medium- and long-term cycles (as well as the corresponding cycles of Juglar, Marx, Kuznets, and Kondratiev) – to the dynamics of capital investments and the renewal of the main productive capital.

The results of critical analysis of the methodological basis of modeling the reproduction process cyclicity in the agrarian sector of the economy substantiated the use of periodogram analysis, Stokes integrals, and Buys-Ballot and autocorrelation functions for the estimation of hidden periodicity in discrete time-series. On the basis of these methods, the analysis of the dynamics of the share of capital investments in gross value added in Ukraine for 1956-2017 determined the duration of long-, medium-, short-term cycles and micro-cycles in both the general reproduction process and in the agrarian sector of the economy. The obtained durations of cycles are statistically significant, closely correlate with the results of studies of other scientists, which confirm their reliability.

Aimar, Th., Bismans, Fr. & Diebolt, C. (2016). Business Cycles in the Run of History. London, Springer.

Allais, M. (1998). Economie et intérêt. Paris: Clément Juglar.

Antomonov, Yu. G. (1983). Methods of Mathematical Biology. Vol. 8. Methods of Analysis and Synthesis of Biological Control Systems. Kyiv: Vyshcha Shkola.

Buys-Ballot, C. H. D. (1847). Les changements périodiques de température, dépendants de la nature du soleil et de la lune, mis en rapport avec le pronostic du temps, déduits d'observations néerlandaises de 1729 à 1846. Utrecht : Kemink & Fils.

Filin, S. A. (2015). Theoretical Bases of Economic Cycle and Management in a Crisis. Moscow: RuScience.

Frisch, R. & Holme, H. (1935). The Characteristic Solutions of Mixed Difference and Differential Equation Occurring in Economic Dynamics. Econometrica, 3(2), 219-225.

Hutorov, A. A. & Pogorelov, A. S. (2006). Analysis of Methods for Detecting of Hidden Periodicities. Herald of Kharkiv National Agrarian University named after V. V. Dokuchaiev “Economics of Agro-Industrial Complex and Nature Management”, 8, 84-89.

Hutorov, A. A. (2009). Application of the Hidden Periodicity Revealing’s Methods in Modelling and an Estimation of Economic Risks. Modelling and Analysis of Safety and Risk in Complex Systems : Proceeding of the Ninth International Scientific School MA SR - 2009 (Saint-Petersburg, July 7-11, 2009). Saint-Petersburg: Saint-Petersburg State University of Aerospace Instrumentation Press, 227-233.

Hutorov, A. O. (2017). Neoindustrial Principles for the Development of Integration Relations in the Agrarian Sector of Economy. Business Inform, 8, 183-191.

Jenkins, G. M. & Watts, D. G. (1968). Spectral Analysis and Its Applications. San Francisco: Holden-Day.

Juglar, C. (1862). Des Crises commerciales et leur retour périodique en France, en Angleterre et aux États-Unis. Paris: Guillaumin et C.

Kalecki, M. (1935). A Macrodynamic Theory of Business Cycles. Econometrica, 3(3), 327-344.

Keynes, J. M. (2012). The General Theory of Employment, Interest and Money. New York: Cambridge University Press.

Kitchin, J. (1923). Cycles and Trends in Economic Factors. The Review of Economics and Statistics, 5(1), 10-16.

Kondratiev, N. D. (2002). Large Conjuncture Cycles and Prediction Theory. Moscow: Ekonomika.

Kufenko, V. (2016). Business Cycles and Institutions: Empirical Analysis. Kumulative Dissertation zur Erlangung des akademischen Grades Dr. oec. Hohenheim: Universität Hohenheim.

Kuznets, S. S. (1930). Secular Movements in Production and Prices. Their Nature and Their Bearing Upon Cyclical Fluctuations. Boston: Houghton Mifflin Co.

Marx, K. (1969). Capital: A Critique of Political Economy. Vol. I, Book I. The Process of Production of Capital. Moscow: Politizdat.

Oliinyk, O. V. (2005). Cyclicity of the Reproductive Process in Agriculture. Kharkiv: Kharkiv National Agrarian University named after V. V. Dokuchaiev Press.

Podlesnaya, V. G. (2017). Logical and Historical Bases of Socio-Economic Cycles Forming. Kyiv: Institute for Economics and Forecasting of NAS of Ukraine Press.

Pustovoit, O. V. (2016). Institutional Nature of Economic Cycles. Case Study from Ukraine. Kyiv: Institute for Economics and Forecasting of NAS of Ukraine Press.

Rastyannikov, V. G. & Deryugina, I. V. (2009). Grain Crop Productivity in Russia. 1795–2007. Moscow: Institute of Oriental Studies.

Ricardo, D. (2001). On the Principles of Political Economy and Taxation. Kitchener: Batoche Books.

Schumpeter, J. (1939). Business Cycles: a Theoretical, Historical, and Statistical Analysis of the Capitalist Process. New York: McGraw-Hill.

Schuster, A. (1898). On the Investigation of Hidden Periodicities with Application to a supposed 26 day Period of Meteorological Phenomena. Terrestrial Magnetism, 3(1), 13-41.

Serebrennikov, M. G. & Pervozvanskiy, A. A. (1965). Detection of Hidden Periods. Moscow: Nauka.

Slutzky, E. (1937). The Summation of Random Causes as the Source of Cyclic Processes. Econometrica, 5(2), 105-146.

Sokolov, I. D., Tarapatov, I. F. & Borysenko, O. P. (1996). Dynamics of Grain Crops Production and Its Forecast. Herald of Agrarian Science, 9, 41-43.

Tugan-Baranovsky, M. I. (1914). Periodic Industrial Crises. History of English Crises. The General Theory of Crises. Saint-Petersburg: O. N. Popova Press.

Zagaytov, I. B. (2011). Laws and Regularities of the Cyclical Nature of Reproduction. Voronezh: Voronezh State Agrarian University Press.

1. Department of Organization Management and Public Administration. National Scientific Center “Institute of Agrarian Economics”. Leading Scientific Researcher, Doctor of Economic Sciences. Kyiv, Ukraine. Contact e-mail: Gutorov.Andrew@gmail.com

2. Department of Management and Administration. Kharkiv National Agrarian University named after V. V. Dokuchaiev. Professor, PhD in Economics. Kharkiv, Ukraine. Contact e-mail: Gutorova.Elena@gmail.com

3. CEO. National Scientific Center “Institute of Agrarian Economics”. Professor, Doctor of Economic Sciences. Kyiv, Ukraine. Contact e-mail: Lupenko@iae.kiev.ua

4. Department of Economics and Social Sciences. Simon Kuznets Kharkiv National University of Economics. Assistant Professor, PhD in Economics. Kharkiv, Ukraine. Contact e-mail: Oleksii.Yermolenko@hneu.net

5. Department of Management. Poltava State Agrarian Academy. Assistant Professor, PhD in Economics. Poltava, Ukraine. Contact e-mail: 8882888@ukr.net